The bending of light rays as they enter a transparent medium—what today is called Snell’s Law—has had a long history of independent discoveries and radically different approaches.  The general problem of refraction was known to the Greeks in the first century AD, and it was later discussed by the Arabic scholar Alhazan.  Ibn Sahl in Bagdad in 984 AD was the first to put an accurate equation to the phenomenon.  Thomas Harriott in England discussed the problem with Johannes Kepler in 1602, unaware of the work by Ibn Sahl.  Willebrord Snellius (1580–1626) in the Netherlands derived the equation for refraction in 1621, but did not publish it, though it was known to Christian Huygens (1629 – 1695).  René Descartes (1596 – 1650), unaware of Snellius’ work, derived the law in his Dioptrics, using his newly-invented coordinate geometry.  Christiaan Huygens, in his Traité de la Lumière in 1678, derived the law yet again, this time using his principle of secondary waves, though he acknowledged the prior work of Snellius, permanently cementing the shortened name “Snell” to the law of refraction. Snell’s Law is a special case of the Eikonal Equation and is useful to calculate the caustic envelope of rays transmitted through undulating surfaces.

Through this history and beyond, there have been many approaches to deriving Snell’s Law.  Some used ideas of momentum, while others used principles of waves.  Today, there are roughly five different ways to derive Snell’s law.  These are:

            1) Huygens’ Principle,

            2) Fermat’s Principle,

            3) Wavefront Continuity

            4) Plane-wave Boundary Conditions, and

            5) Photon Momentum Conservation.

The approaches differ in detail, but they fall into two rough categories:  the first two fall under minimization or extremum principles, and the last three fall under continuity or conservation principles.

Snell’s Law: Huygens’ Principle

Huygens’ principle, published in 1687, states that every point on a wavefront serves as the source of a spherical secondary wave.  This was one of the first wave principles ever proposed for light (Robert Hooke had suggested that light had wavelike character based on his observations of colors in thin films) yet remains amazingly powerful even today.  It can be used not only to derive Snell’s law but also properties of light scattering and diffraction.  Huygens’ principle is a form of minimization principle:  it finds the direction of propagation (for a spherically expanding wavefront from a point where a ray strikes a surface) that yields a minimum angle (tangent to the surface) relative to a second source.  Finding the tangent to the spherical surface is a minimization problem and yields Snell’s Law.

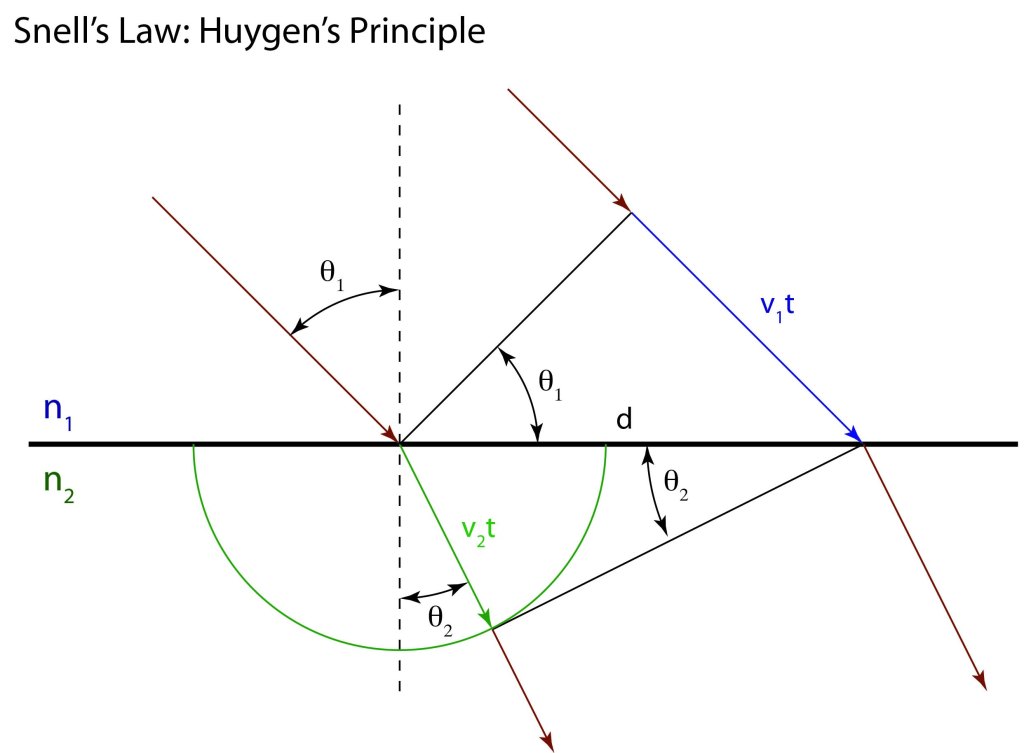
            The use of Huygen’s principle for the derivation of Snell’s Law is shown in Fig. 1.  Two parallel incoming rays strike a surface a distance d apart.  The first point emits a secondary spherical wave into the second medium.  The wavefront propagates at a speed of v2 relative to the speed in the first medium of v1.  In the diagram, the propagation distance over the distance d is equal to the sine of the angle

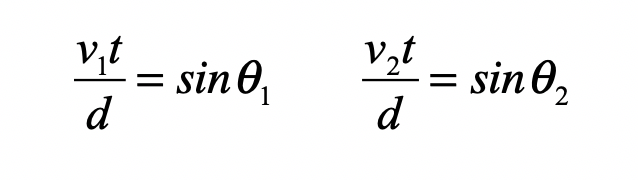
Solving for d and equating the two equations gives

The speed depends on the refractive index as

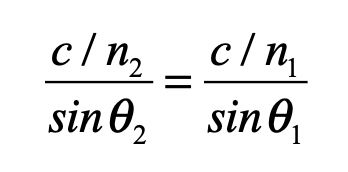
which leads to Snell’s Law:

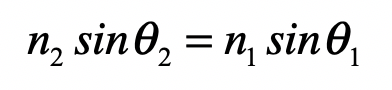
Snell’s Law: Fermat’s Principle

Fermat’s principle of least time is a direct minimization problem that finds the least time it takes light to propagate from one point to another.  One of the central questions about Fermat’s principle is: why does it work?  Why is the path of least time the path light needs to take?  I’ll answer that question after we do the derivation.  The configuration of the problem is shown in Fig. 2.

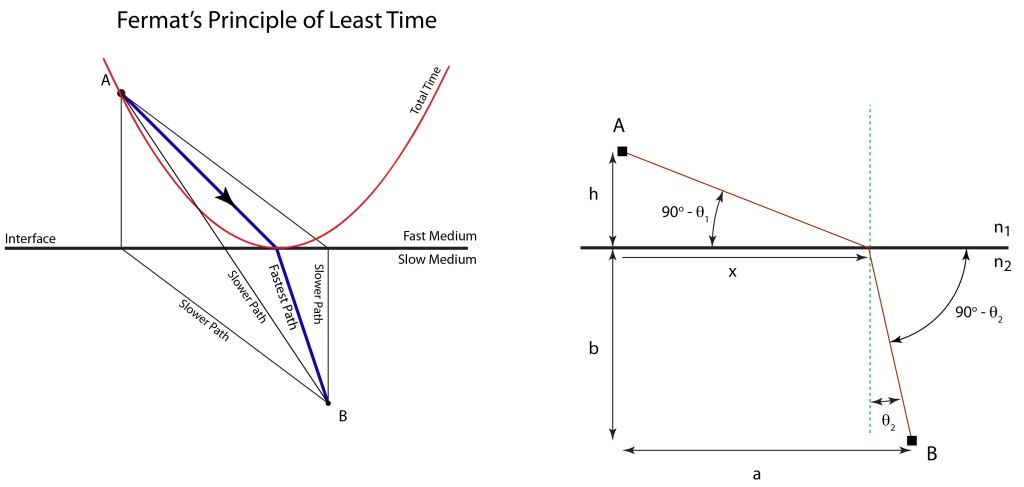
Consider a source point A and a destination point B.  Light travels in a strait line in each medium, deflecting at the point x on the figure.  The speed in medium 1 is c/n₁, and the speed in medium 2 is c/n₂.  What position x provides the minimum time?

The distances from A to x, and from x to B are, respectively:

The total time is

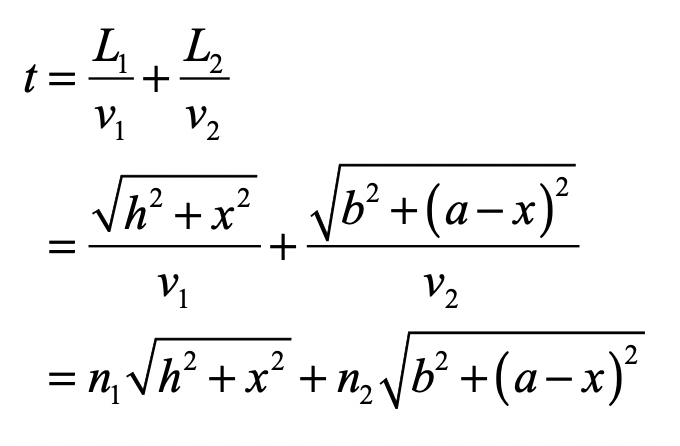
Minimize this expression by taking the derivative of the time relative to the position x and setting the result to zero

Converting the cosines to sines yields Snell’s Law

Fermat’s principle of least time can be explained in terms of wave interference.  If we think of all paths being taken by propagating waves, then those waves that take paths that differ only a little from the optimum path still interfere constructively.  This is the principle of stationarity and is related to the principle of least action.  The time minimizes a quadratic expression that deviates from the minimum only in second order (shown in the right part of Fig. 2).  Therefore, all “nearby” paths interfere constructively, while paths that are farther away begin to interfere destructively.  Therefore, the path of least time is also the path of stationary time and hence stationary optical path length and hence the path of maximum constructive interference.  This is the actual path taken by the wave—and the light.

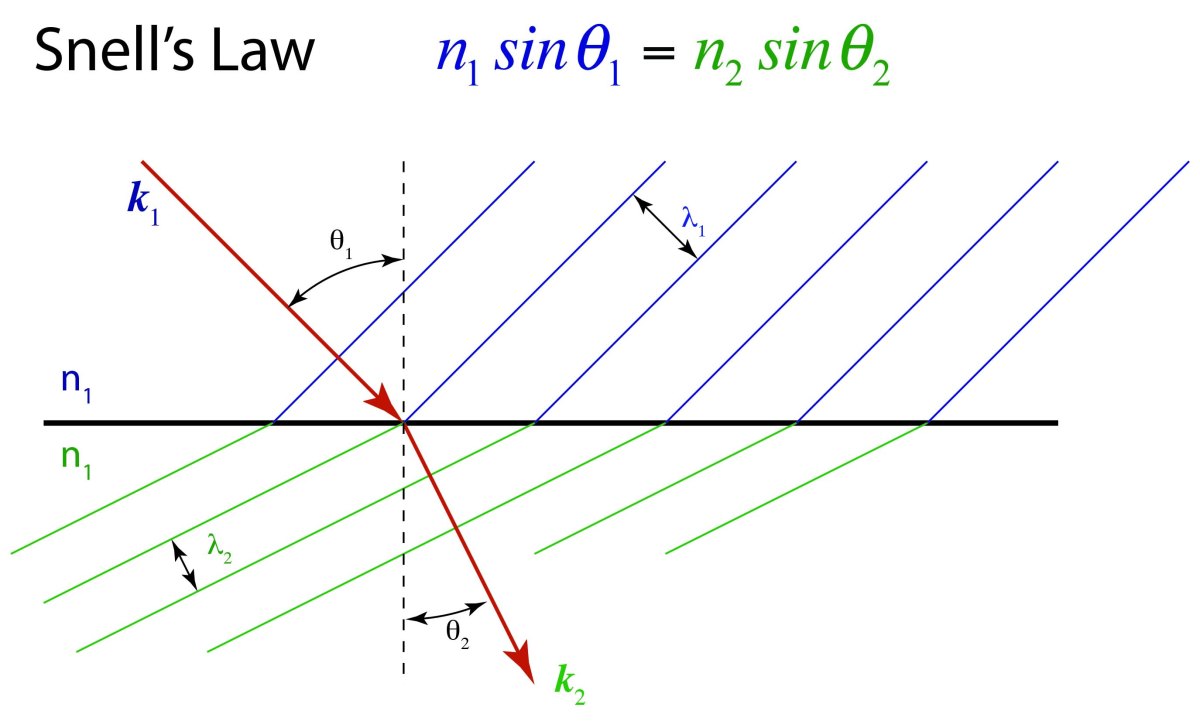
Snell’s Law: Wavefront Continuity

When a wave passes across an interface between two transparent media the phase of the wave remains continuous.  This continuity of phase provides a way to derive Snell’s Law.  Consider Fig. 3.  A plane wave with wavelength l₁ is incident from medium 1 on an interface with medium 2 in which the wavelength is l₂.  The wavefronts remain continuous, but they are “kinked” at the interface. 

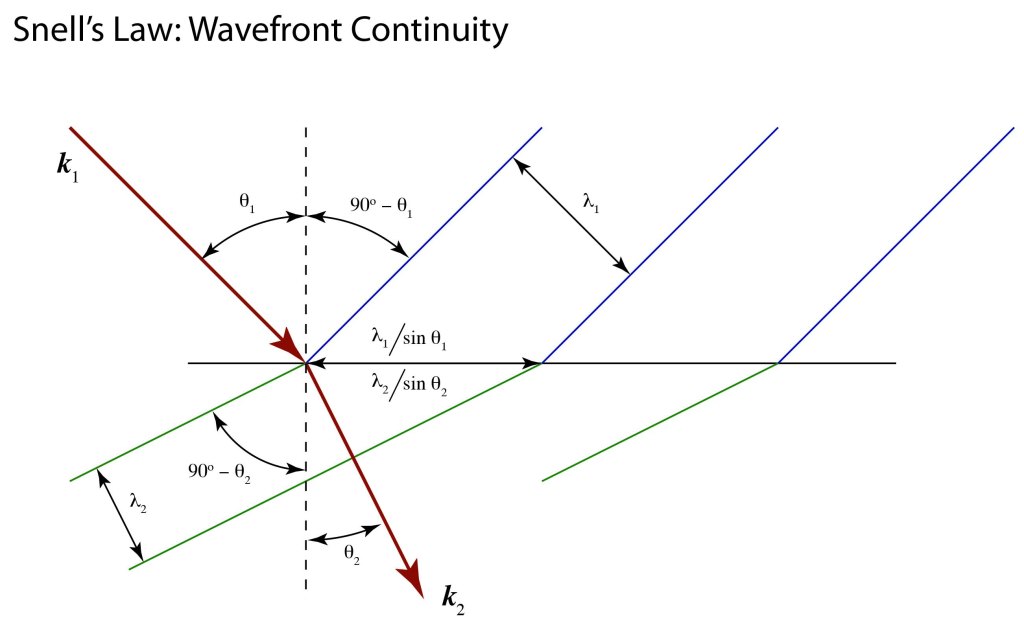
The waves in medium 1 and medium 2 share the part of the interface between wavefronts.  This distance is

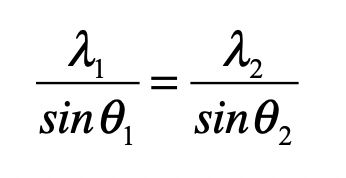
The wavelengths in the two media are related to the refractive index through

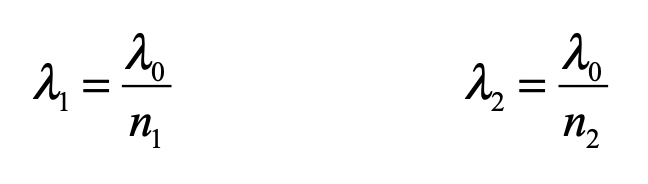
where l₀ is the free-space wavelength.  Plugging these into the first expression yields

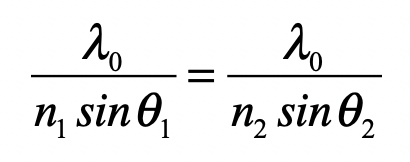
which relates the denominators through Snell’s Law

Snell’s Law: Plane-Wave Boundary Condition

Maxwell’s four equations in integral form can each be applied to the planar interface between two refractive media.

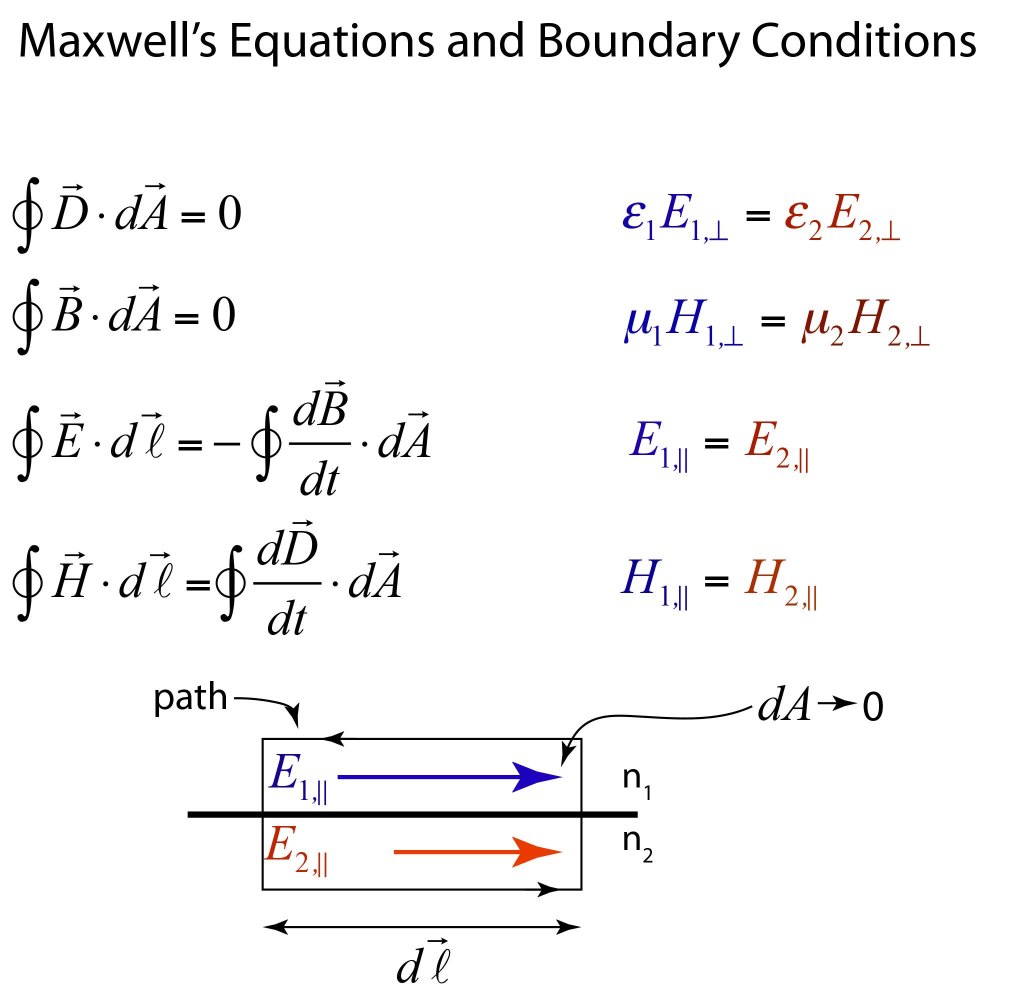
All four boundary conditions can be written as

where i, r and t stand for incident, reflected and transmitted. The only way this condition can be true for all possible values of the fields is if the phases of the wave terms are all the same (phase-matching), namely

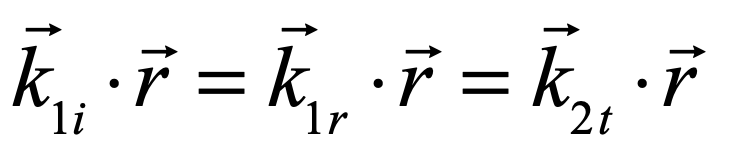
which in turn guarantees that the transverse projection of the k-vector is continuous across the interface

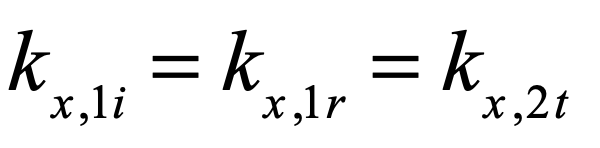
and the transverse components (projections) are

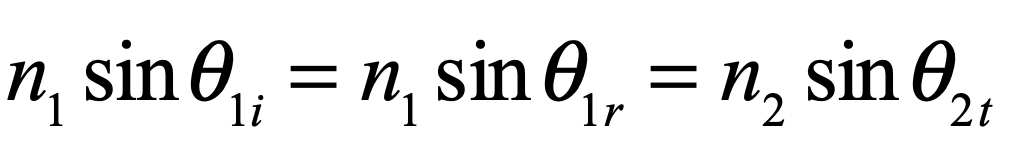
where the last line states both Snell’s law of refraction and the law of reflection. Therefore, the general wave boundary condition leads immediately to Snell’s Law.

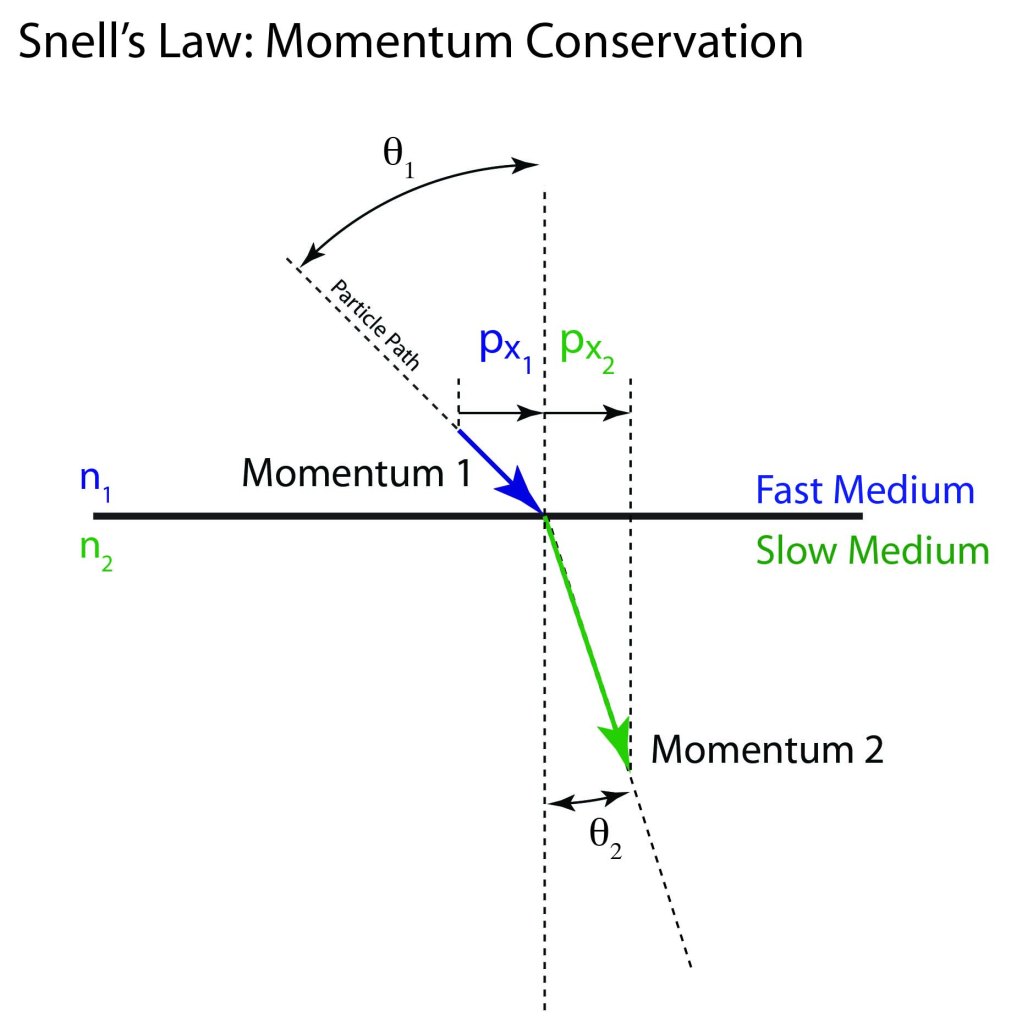
Snell’s Law: Momentum Conservation

Going from Maxwell’s equations for classical fields to photons keeps the same mathematical form for the transverse components for the k-vectors, but now interprets them in a different manner.  Where before there was a requirement for phase-matching the classical waves at the interface, in the photon picture the transverse k-vector becomes the transverse momentum through de Broglie’s equation

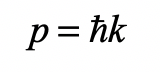
Therefore, continuity of the transverse k-vector is interpreted as conservation of transverse momentum of the photon across the interface.  In the figure the second medium is denser with a larger refractive index n2 > n1.  Hence, the momentum of the photon in the second medium is larger while keeping the transverse momentum projection the same.  This simple interpretation gives the same mathematical form as the previous derivation using classical boundary conditions, namely

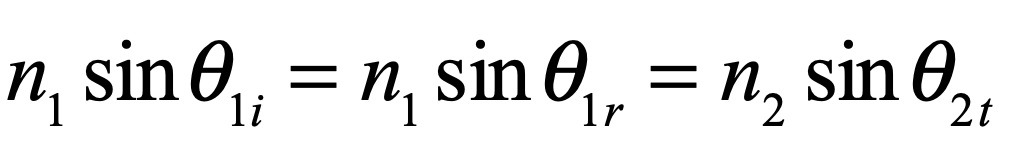
which is again Snell’s law and the law of reflection.

Recap

Snell’s Law has an eerie habit of springing from almost any statement that can be made about a dielectric interface. It yields the path of least time, tracks the path of maximum constructive interference, produces wavefronts that are extremally tangent to wavefronts, connects continuous wavefronts across the interface, conserves transverse momentum, and guarantees phase matching. These all sound very different, yet all lead to the same simple law of Snellius and Ibn Sahl.

This is deep physics!

---

---

New from Oxford University Press: Interference and the History of Light and Optics (2023)